A dollar today is worth more than tomorrow thanks to inflation and compound growth. The former means money will buy more now relative to any point in the future. The latter means capital also has more investment potential at present than it will tomorrow.
There’s a lot to unpack here. Bear with me and keep reading, though. By the end of this article, everything will be crystal clear.
Diving deeper into why a dollar today is worth more than tomorrow
Would you rather receive $500,000 today or $1 million at age 65?
Many people would intuitively choose the million. I mean it’s literally double the money, right? If you’re a typical earner, saving an extra $500,000 could take decades of pinching pennies. In this scenario, you’d just have to wait!
Here’s the thing, though. Depending on how old you are, $500,000 today could be a far better deal. Let’s look at those aforementioned concepts – compound interest and inflation – to understand why.
How compound interest makes a dollar today worth more than tomorrow
As of writing, I’m 24. If I invested $500,000 in the stock market right now, it would grow to an estimated $24,892,590 by the time I’m 65 (41 years later). This assumes the stock market returns its historical average of around 10% annually.
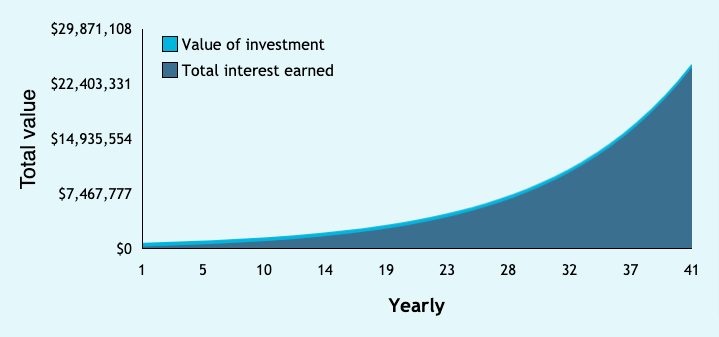
Suddenly, $1 million doesn’t sound all that exciting.
In fact, agreeing to take a million at 65 doesn’t make mathematical sense until you’re roughly 58. At that point, there isn’t enough time for compound growth to turn your $500,000 into more than $1 million by age 65 (seven years later).
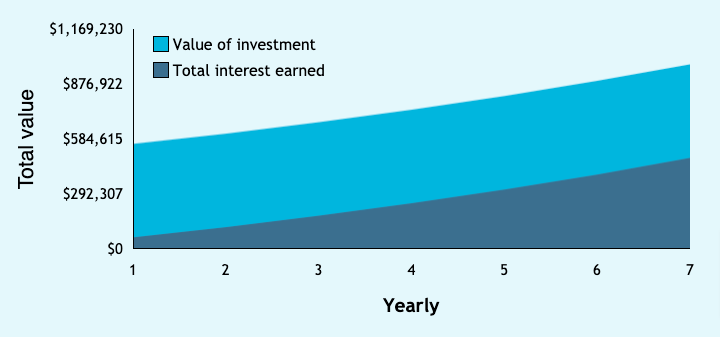
Do you notice a difference between the shapes of these graphs, by the way? The first is a dramatic curve upward while the second seems to grow linearly.
In reality, the second graph is just a slice of the first.

This visualization is crucial for understanding why a dollar today is worth more than tomorrow.
Having money to invest right now lets you tap into a longer compound growth curve than you’ll ever be able to again. If your timeline is long enough, this can make a dollar today worth more than even two down the road.
Conversely, lacking the ability (or willingness) to invest today severely limits your money’s growth potential. The longer you wait, the more difficult it is to catch up.
How inflation makes a dollar today worth more than tomorrow
Thanks to inflation, a dollar buys more today than it will at any point in the future.
For example, $500,000 would have been more than enough to purchase a detached house in Toronto 10 years ago. In fact, you would’ve been able to choose from countless homes for that money.
Today? You’d struggle to find one-bedroom condos with asking prices that low. Average home valuations in the city have surpassed $1 million according to Zolo.
Waiting won’t really help you, either. By 2026, average house prices in Toronto are expected to surpass $3.5 million.
In other words, accepting $500,000 today would almost invariably be better than waiting for $1 million at 65 if you’re looking to buy real estate in Toronto. This is probably true in any major North American market, for that matter.
Even if you’re 60 right now, waiting five years would only double your money whereas the average house price will more than triple in that same time.
Once again, a dollar today is worth more than two down the road.
Of course, inflation affects much more than just real estate in Toronto. Throughout the world, virtually everything becomes more expensive over time. Check out the Consumer Price Index for a breakdown of how various costs (i.e. food and energy) inflate over time in America.
The bottom line? A dollar’s value in regards to its most basic function – buying things – is quite literally higher today than it will be tomorrow.
Bonus: How risk makes a dollar worth more today than tomorrow
So far, we’ve assumed that either option – $500,000 today or $1 million at 65 – is guaranteed. Real life doesn’t work like that, though. Often, waiting longer to collect your money increases the chance whoever promised it might not honor their commitment.
Let’s say you’re a business executive. Your company’s board is offering a $500,000 severance package today. Alternatively, you can stick with the company until 65 and receive $1 million.
Waiting would carry many risks. Will the company still exist when you’re 65? Will it still be in a position to make that payment?
Additionally, what if your value as an executive increases substantially between now and then? You’d be locked into accepting $1 million when you could’ve done better elsewhere.
If $500,000 is a fair severance package based on your situation today, accepting it would be justifiable in light of these risks.
To give a more relatable example, this rationale explains why you’d often be better off asking for a raise today than waiting.
Even if you believe a bigger raise would be possible down the road, there’s no guarantee. Conditions beyond your control (*cough* like a global pandemic *cough*) could force the company to scale back. At that point, you’d regret not having asked for a raise when times were good.
To phrase this differently, money in your pocket today is a sure thing. Tomorrow? Not so much.
Understanding the time value of money formula
The time value of money is a concept experts use to sum up – and quantify – the concepts we’ve discussed so far. With it, you can also identify scenarios in which a dollar today isn’t worth more than tomorrow and you’d be better off waiting.
The time value of money formula revolves around these variables:
- FV: Future value
- PV: Present value
- i: Annual interest rate
- n: Number of compounding periods in a year
- t: Number of years
Next, let’s look at how these variables come together in two common scenarios.
Scenario #1: Starting with a known sum of money today and determining its future value
In this scenario, FV is the answer we’re looking for. As such, here’s our formula:
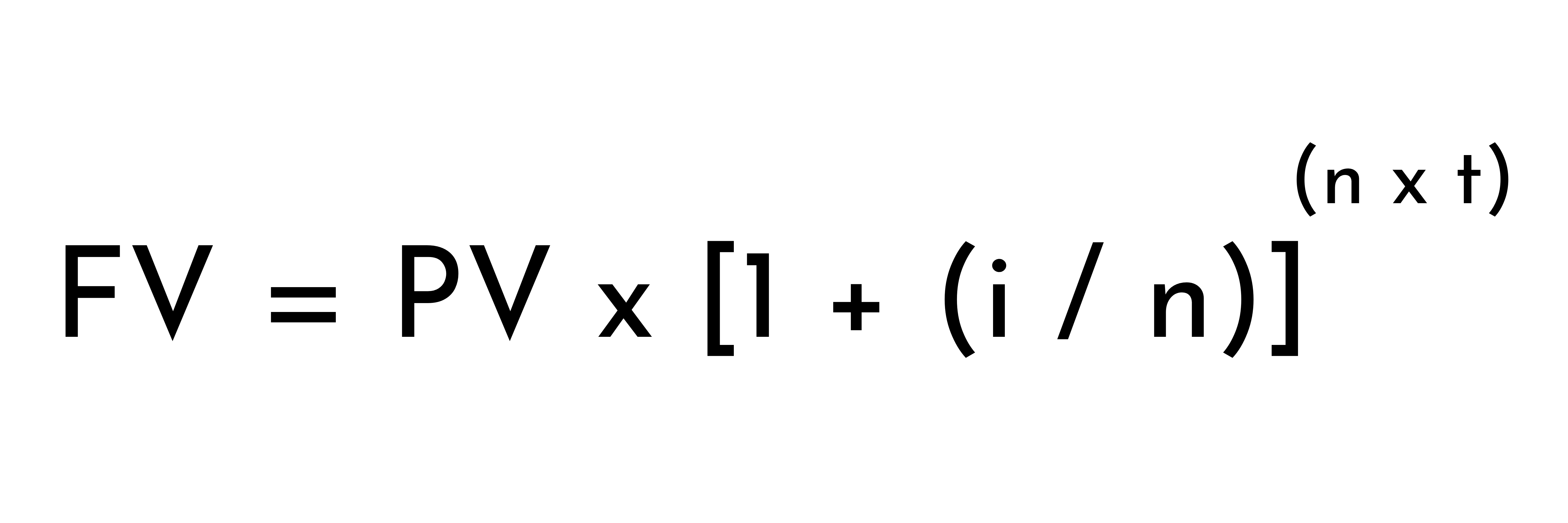
Think back to my earlier example in which we discussed whether to accept $500,000 today or $1 million at 65. Any conditions under which FV ends up being higher than $1 million would theoretically warrant accepting $500,000 rather than waiting.
Here’s what my inputs would look like as a 24-year-old investing $500,000 in the U.S. stock market (10% average return compounding once annually) until 65 (41 years from now):
- PV: $500,000
- i: 10%
- n: 1
- t: 41
Here’s what those elements look like in place (along with the answer):
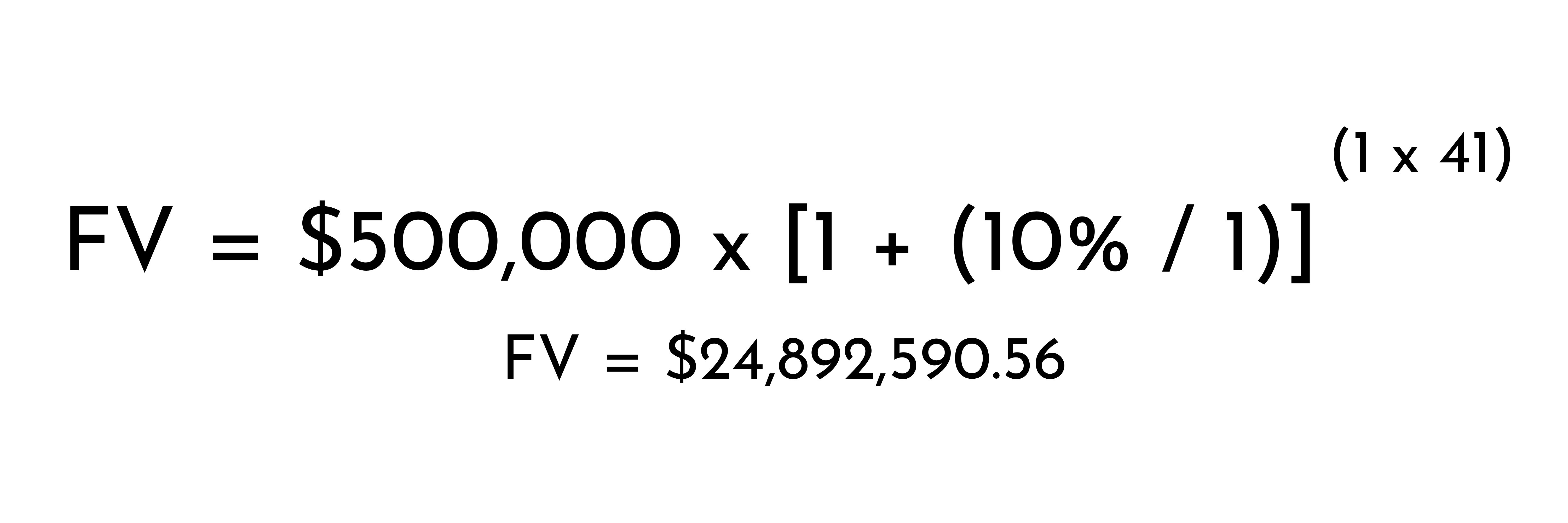
Conversely, here’s what the time value of money formula would look like if I were a 58-year-old deciding whether to invest $500,000 in U.S. stocks until 65 (7 years from now) rather than waiting to receive $1 million:
- PV: $500,000
- i: 10%
- n: 1
- t: 7

As you can see, our formula confirms it would make sense to accept $500,000 at 24 because FV ends up being far greater than the $1 million we’d receive by waiting. At 58, however, the FV would end up being less than $1 million. As such, we’re theoretically better off waiting.
Practical use cases for this forward-looking time value of money formula
We all – yes, even those of us who never have to choose between receiving $500,000 today or $1 million at 65 – make decisions in which the time value of money formula can be useful. Here are some examples.
Calculating opportunity costs
An opportunity cost is a financial loss you incur by choosing one option over another. For example, let’s say you’re deciding whether to visit Spain or invest that money in the stock market. Your opportunity cost would be the investment returns, which you could calculate using the above formula. A vacation costing $5,000 might produce these inputs:
- PV: $5,000
- i: 10%
- n: 1
- t: 20
Note: Time (t) would simply be however long you’d keep the money invested. I put 20 years above.
The result:
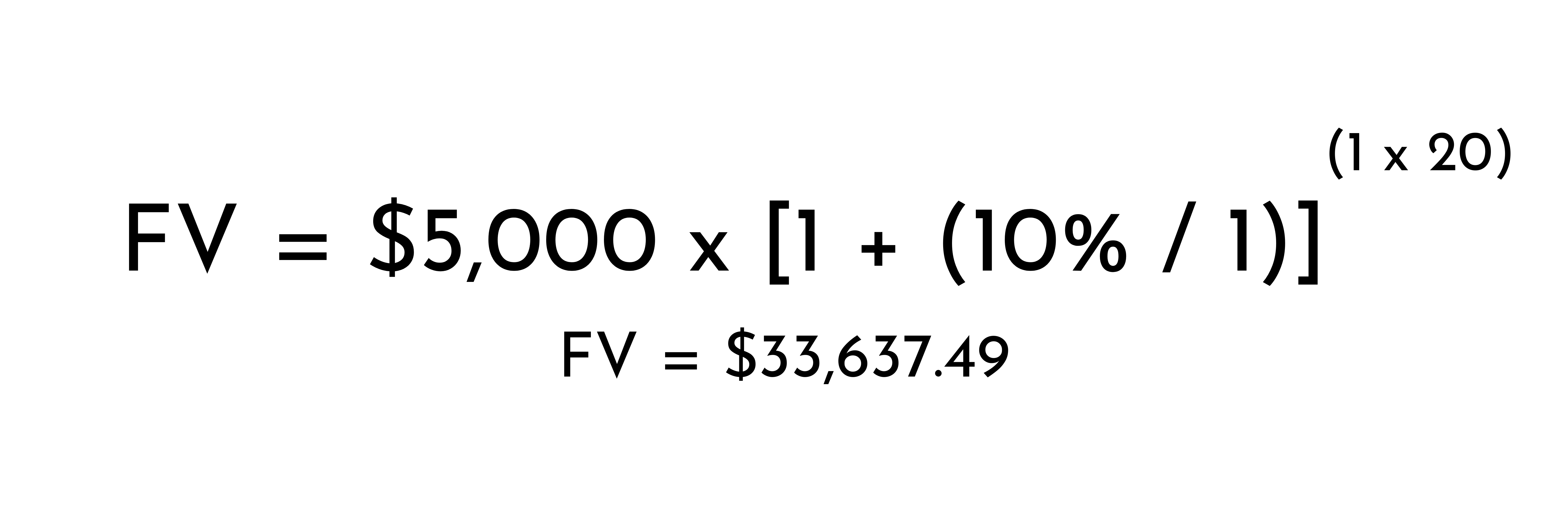
Your vacation’s opportunity cost would be $33,637 in this scenario.
As a side note, that doesn’t mean you shouldn’t travel. It’s very possible taking a vacation to Spain right now will benefit you more than having an extra $33,637 in 20 years. It’s helpful to calculate any decision’s underlying opportunity cost, however, since you can then compensate for it later. More on this shortly.
Estimating your retirement fund’s future size
You could also use this forward-looking time value of money formula to project how your retirement account will grow in the future.
For example, let’s say you currently have a balance of $100,000. With 20 years to go until retirement, your calculations might look like this:
- PV: $100,000
- i: 10%
- n: 1
- t: 20
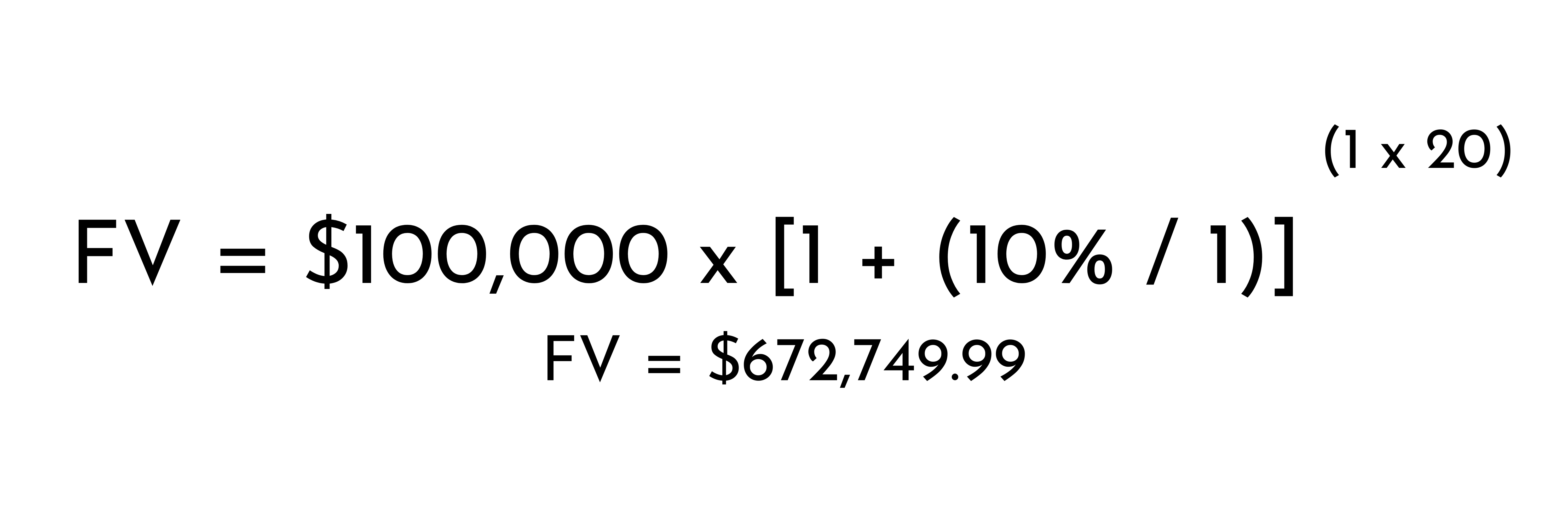
You could subsequently compare that estimated balance ($672,749) to your expected living costs in retirement and decide how aggressively you need to save going forward.
Scenario #2: Starting with a future value in mind and calculating how much you’d need now to stay on pace
In this scenario, PV is the answer we’re looking for. As such, this is the appropriate formula:
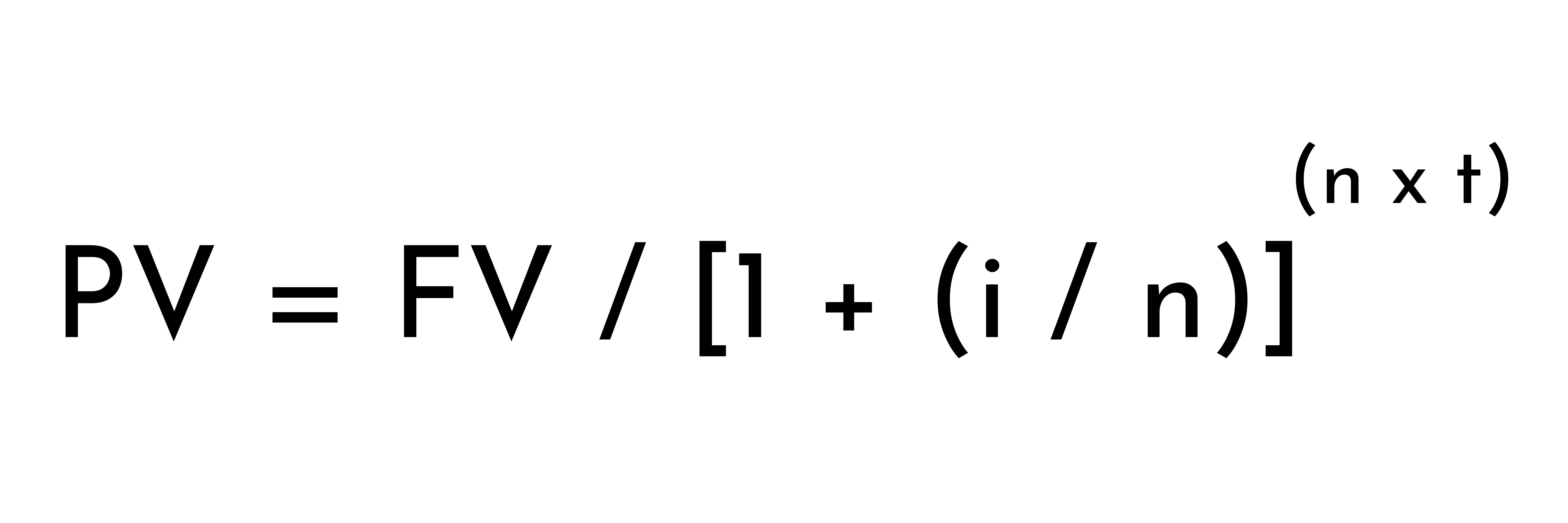
With this formula, we could calculate just how much money we’d need to invest at 58 to make up for having turned down $500,000 at 24. Here are the inputs:
- FV: $24,892,590
- i: 10%
- n: 1
- t: 7
Here are the elements in place with the answer below:
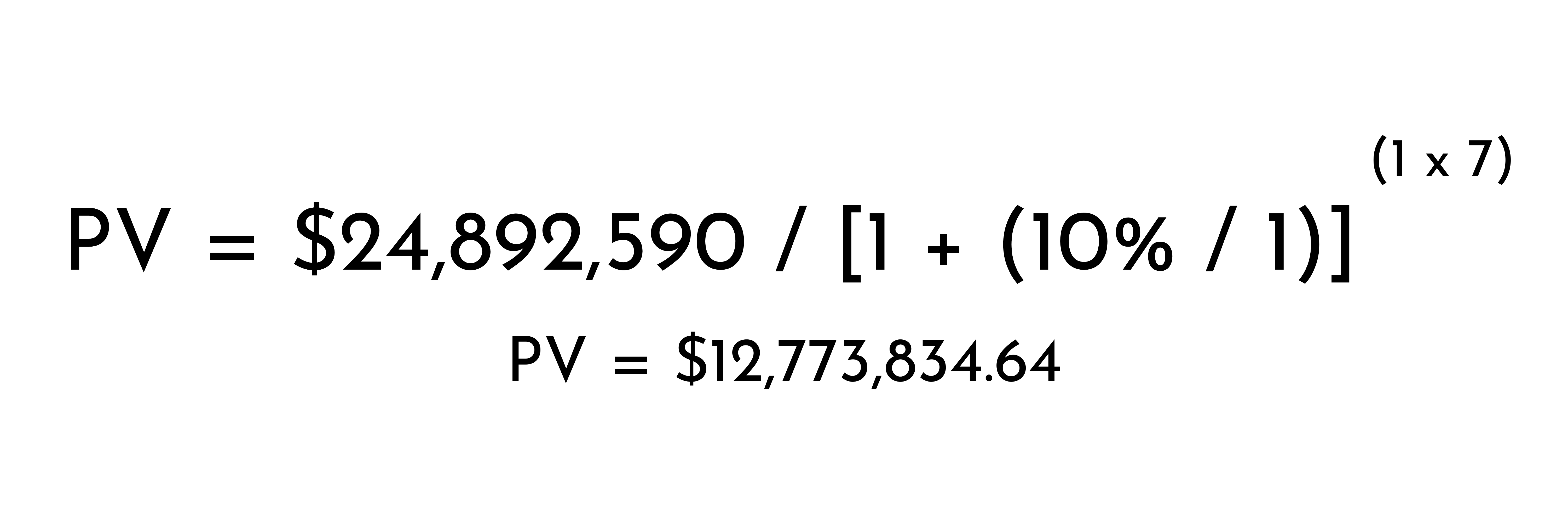
As you can see, choosing to receive $1 million at 65 instead of $500,000 at 24 wouldn’t actually double your reward in meaningful terms. It would place you nearly 13 times farther behind.
Practical use cases for this backward-looking time value of money formula
Once again, the aforementioned scenario is great for visualizing this backward-looking time value of money formula but doesn’t have much direct practical value. Here are some more useful examples.
Determining whether you’re on pace to hit your financial independence number
Your financial independence number is exactly what it sounds like – a certain amount of money you could obtain and promptly retire. To find this number, many people simply multiply their annual spending by 25. Check out this article explaining that calculation’s significance and how you can tailor it to your situation.
However you arrive at your financial independence number, the next logical step would be to determine whether you’re currently on pace to hit it. In other words, do you have enough money currently invested to achieve financial independence by a reasonable age? If not, how far off are you? Our formula provides the answers.
For example, let’s say your financial independence number is $2 million and you’re 24 with plans to retire at 65. Here’s what your inputs might look like, assuming you’ll be investing in the U.S. stock market:
- FV: $2,000,000
- i: 10%
- n: 1
- t: 41
The result:

In plain English, having $40,172 invested at 24 would put you on pace to hit your financial independence number of $2 million by 65, assuming the market returns 10% on average each year.
Allocating your resources efficiently
If the backward-looking time value of money formula reveals you’re well ahead of schedule for a specific goal like financial independence, that may be your cue to shift priorities. For example, you could comfortably make decisions that carry higher opportunity costs than would be sensible for someone who’s further behind.
This is how I’ve personally planned my finances over the next few years. Based on my calculations, I need roughly $500,000 invested at 30 to achieve the base level of financial independence I’d like by 50. My math looks like this:
- FV: $1,500,000
- i: 5.56% (chosen as a conservative estimate of my portfolio’s average annual return)
- n: 1
- t: 20
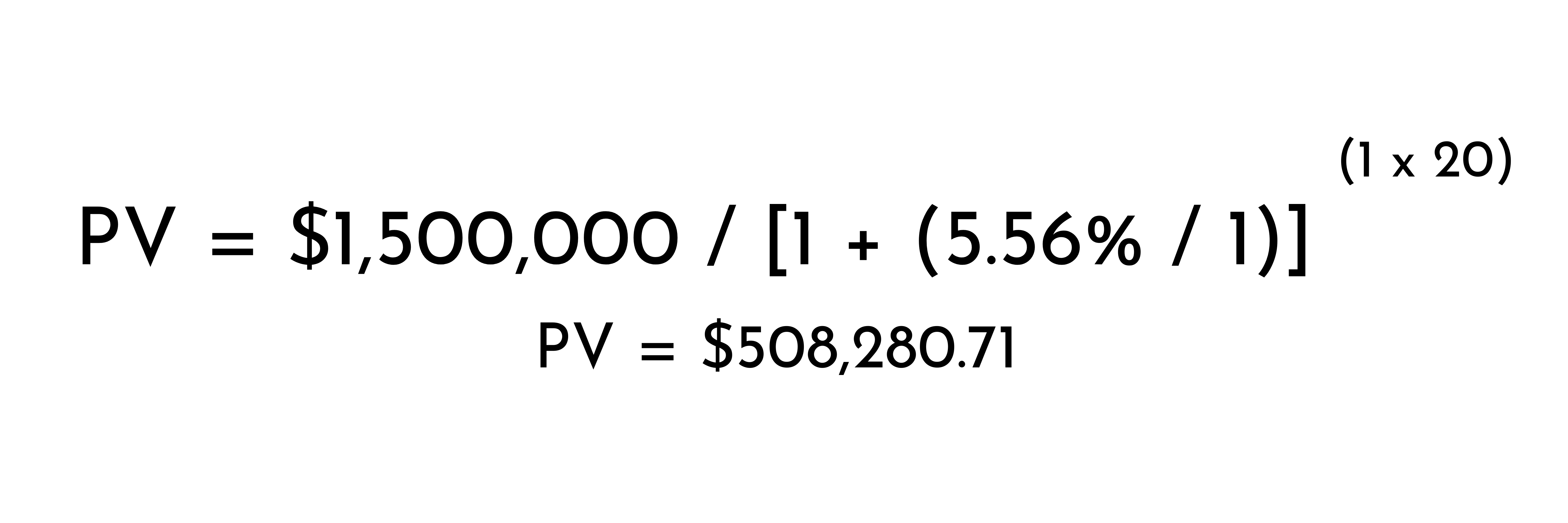
Past the $500,000 mark, I’ll be more comfortable spending money discretionarily and purchasing a primary residence in my ideal location (somewhere remote in which my true equity – net of expenses – is unlikely to rise on pace with stocks for the long haul, representing a substantial opportunity cost).
Pro-tip: Try not to overthink the math
This might seem ironic given I’ve just spent the last several sections crunching numbers. However, it’s worth pointing out personal finance is about much more than just math or even maximizing every dollar’s potential for growth.
In fact, very financially savvy people often make decisions that incur significant opportunity costs and don’t make sense based on the time value of money formula.
The key is to be conscious of such decisions. Too many people go through life unknowingly stomping all over their finances then wondering why they’re broke and miserable as if it’s some mystery.
By evaluating every major financial decision through the lens of money’s time value, however, you can avoid this.
Deciding whether you can afford a brand new car? Use the forward-looking time value of money formula to calculate what your retirement account would look like if you bought a used car and invested the difference instead.
Not sure whether upgrading your degree is worth it? Calculate your tuition’s opportunity cost using the forward-looking formula. Compare this to the raise you’d get with your enhanced credentials.
Again, even if the option you ultimately choose isn’t the most financially beneficial, at least you’ll be aware. You can subsequently compensate for it (i.e. by saving more aggressively in the future than you otherwise would have).
I hope this article has been thought-provoking. Feel free to let me know your thoughts on Twitter. Check out more of my personal finance articles here.

